Abacuses and counting machines

Can you count without words?

The simplest machine for counting is your fingers. Counting beads and counting stones can also be found across cultures. Machines for counting show how we can do mathematics without even writing mathematics, and how numbers have transformed society.

It was the abacus and similar counting instruments that made calculations easy, and this made sophisticated trade possible. This paved the way to the development of civilization.

Babylonians, Romans, Aztecs, Incas, Chinese and Indians are just some of the civilizations who have made use of counting machines. Today counting machines are more important than ever. Our computers and phones are just particularly elaborate devices for performing complex calculations. Counting machines have changed the face of culture across the world.

The Chinese Abacaus

The abacus is a cultural icon of Chinese mathematics. This video explains the basics of using a Chinese abacus to add numbers. You can do much more with an abacus and they are an excellent tool for rapid calculation in the hands of a skilled user.

The Sunzi Suanjing

The Sunzi Suanjing ("Master Sun's Mathematical Manual") describes algorithms for arithmetic using rods as a tool for counting. These algorithms are essentially the same as those we use today to perform long division, long multiplication and so forth today. The Sunzi Suanjing also explains how to calculate square roots.

The Sunzi Suanjing contains the earliest known example of the theorem known in the West today as The Chinese Remainder Theorem. The Chinese Remainder Theorem is used today as part of the standard algorithms used to secure messages on the internet.

The Chinese Remainder Theorem was also used in one of the most amazing maths results proved in the twentieth century: Gödel's incompleteness theorem. These results show that there are some mathematical statements that cannot be proved either true or false: maths must always be incomplete. Gödel was born in Brno a city in the present-day Czech republic.

Gödel's theorem provided the inspiration for Alan Turing's work on the limits of computability.

The Analytical Engine

The English mathematician Charles Babbage designed a mechanical machine called the Analytical Engine which would have been the first programmable computer - had it been built at the time! A small part of a simplified was constructed by Babbage before he died and his son took this work further. It was not until 1991 that the London Science Museum built a working specimen of Babbage's "Difference Engine Number 2".

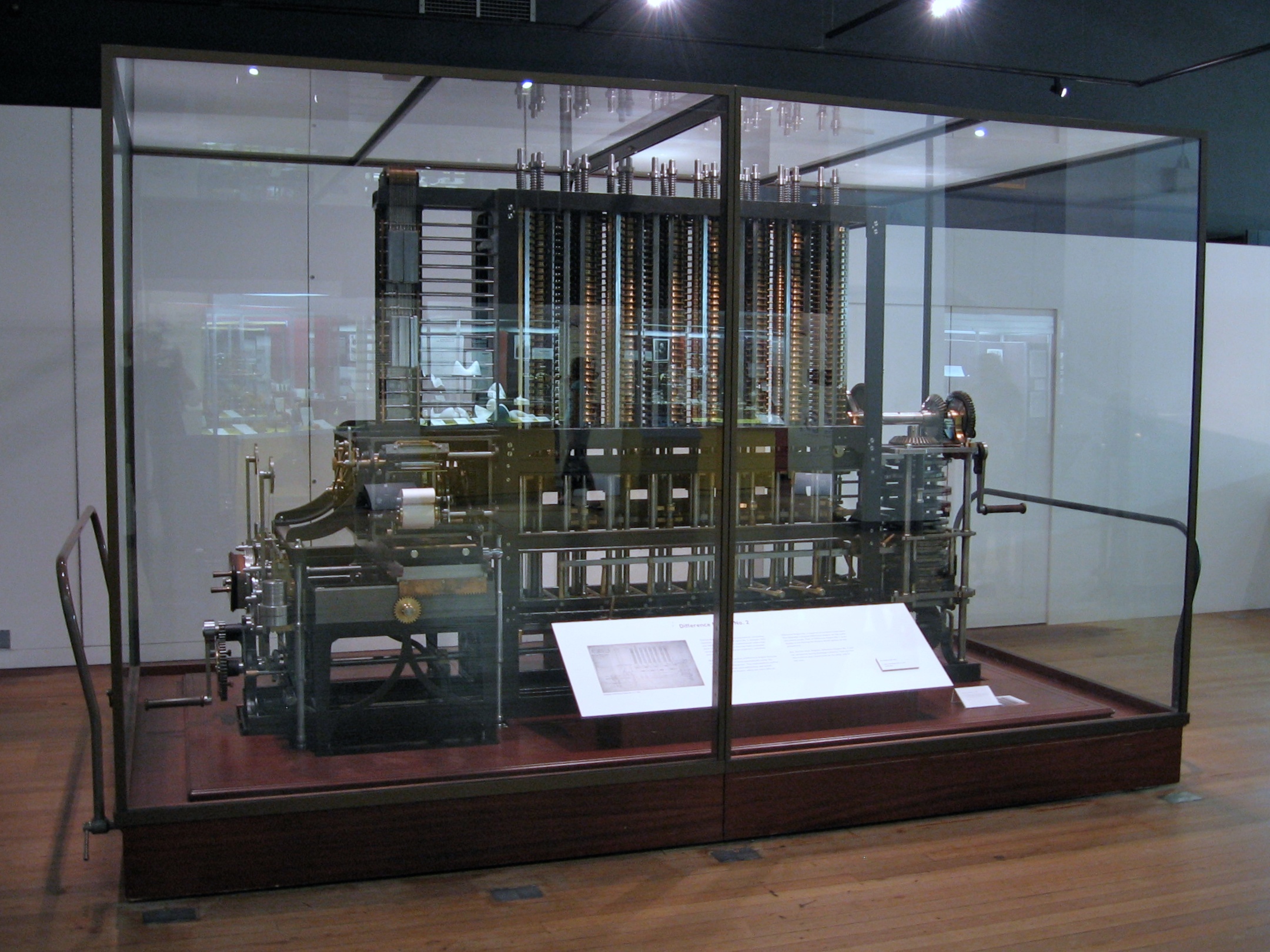
Even though the machine was not built, it was possible for mathematicians to think how they would use it if it was built. Ada Lovelace made notes on how one could calculate "Bernoulli numbers" using the machine. These notes are generally believed to be the first computer program, making Lovelace the first ever computer computer programmer.

The Bernoulli numbers are a sequence of fractions that occur in a number of interesting mathematical problems. They are named after Bernoulli whose work on them was published in 1713 after Bernoulli was dead. They were independently studied by the Japanese mathematician Seki Takakazu, whose work was published in 1712, after Seki was dead. It would perhaps be better to call them Seki-Bernoulli numbers.