The maths and art of spacetime

Challenge: Can you imagine four dimensions?

What is four-dimensional space?

A point in two-dimensional space is determined by two coordinates (x,y). A point in three-dimensional space is determined by three coordinates (x,y,z). So we can define four-dimensional space to be given by points of the form (x,y,z,t).

If you compete in a decathlon or a heptathlon, your result in each event is a number, for example the height you managed in the high-jump and the distance you threw the discus. The result of a decathlon gives you a point in ten-dimensional space. The result of a heptathlon gives you a point in seven-dimensional space.

Analysing big data sets has become very important, and is changing the world. Surprisingly, thinking about the geometry of data sets can be very useful. This is because most of us find it easier to think about space than to think about data, so it helps to use our intuition about geometry to understand data.

For example, there are thousands of stocks on the stock market to invest in, so a bank's investments can be represented as a point in a very high-dimensional space. By thinking in these terms, it turns out that you can find one possible way to balance risk and return in your investments using ideas that you can understand with just pictures.

Time as the fourth dimension

Space-time is an example of a 4-dimensional space. Any event that happens, happens in a particular place and at a particular time. So the data needed to describe an event consists of four numbers (x,y,z,t) where (x,y,z) gives the coordinates in space and t gives the time the event occurred. This is what is meant when people say that time is the fourth dimension.

A time-lapse photograph gives you one way of visualising space-time.

Thinking of space-time as a four-dimensional space is a central idea of Einstein's theory of special relativity. When you switch from one person's viewpoint to another's, the natural set of coordinates for space-time will change. Einstein's big idea was to realise that in the space-time we live in, when you change viewpoints between two people moving at different speeds, the change in coordinates mixes up the space and time coordinates. This must be true to account for the strange fact that two people, whatever speed they are travelling at relative to each other, both measure light as having the same speed.

Surprisingly, to understand special relativity you don't need to picture all four dimensions at once. You can understand the main ideas using 2-dimensional pictures with one space dimension and one time dimension. This graphical approach was developed by the Polish mathematician Hermann Minkowski.

In Minkowski diagrams, one axis is used to represent time and another represents space. This allows you to draw a diagram of the history of all the events that have taken place along a line.

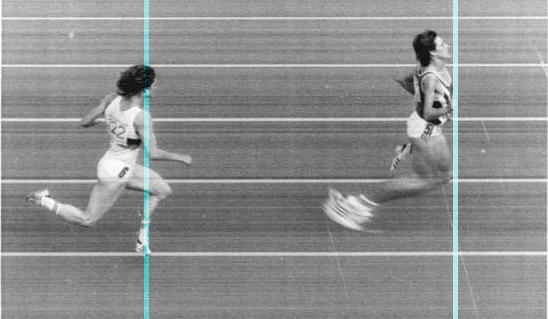
The photo-finish image in an athletics race is a Minokowski diagram. It does not show a picture of the finish at a moment in time. Instead it shows a picture of the one-dimensional line finish line over time.

Each vertical line in the photo-finish photograph is a snapshot of the finish at a moment in line. The blue line on the right in the example below is a snapshot of the of the finish at the time 53.24s at the exact instant Sabine Bush won the world championships as her chest crossed the line. The line on the left in the example is a snapshot of the finish at 53.55s as Cornelia Ulrich crossed the line in second place. The entire photo is made up of thin snapshots of the finish line all taken at slightly different times.

This is why photo-finish pictures always look a little strange, they aren't photos of space they are photos of space-time! The athletes seem to have impossible shapes and slightly curved limbs. This is because their arms and legs keep moving as they cross the line.

Some of the pictures on our chaos theory page show the fourth-dimension by plotting the entire history of the motion of an object, just like a time-lapse photograph.

The fourth-dimension in art

The novel Slaughterhouse 5 by American novelist Kurt Vonnegut is generally agreed to be one of the greatest science-fiction novels written. It is about a man, Billy Pilgrim, who learns to travel through time after he is kidnapped by aliens from the planet Tralfamadore. The book talks about how this knowledge helps him live with the horrors he witnessed when the city of Dresden was firebombed at the end of the second world war. This is the start of Chapter 5:

Billy Pilgrim says that the Universe does not look like a lot of bright little dots to the creatures from Tralfamadore. The creatures can see where each star has been and where it is going, so that the heavens are filled with rarefied, luminous spaghetti. And Tralfamadorians don't see human beings as two-legged creatures, either. They see them as great millepedes - "with babies' legs at one end and old people's legs at the other," says Billy Pilgrim.

The French artist Marcel Duchamp was mocked when his 1912 painting "Nude Descending a Staircase, No. 2" was exhibited at in New York in 1913. One critic described it as resembling "an explosion in a shingle factory". It is now widely regarded as a classic of Modernist painting.

Duchamp later explained the thinking behind his painting:

My aim was a static representation of movement, a static composition of indications of various positions taken by a form in movement—with no attempt to give cinema effects through painting. The reduction of a head in movement to a bare line seemed to me defensible.

There are other ways of thinking of the fourth-dimension. In his painting Crucifixion (Corpus Hypercubus) the Spanish artist Salvador Dali shows the surface a four-dimensional cube (a hypercube), flattened into three dimensions.

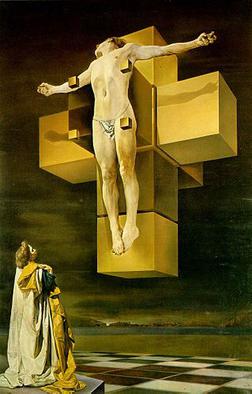
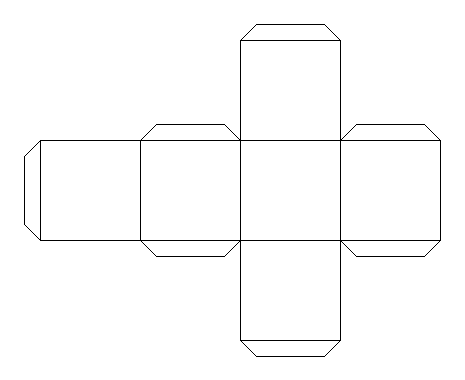
Dali's image represented the surface of a hypercube in much the same way as the flat-pack diagram below represents the surface of a cube.

The Geometry of Four-Dimensional Space

The French philosopher and mathematician René Descartes invented the idea of coordinate geometry, where points on a plane or in space are represented by their coordinates. This allows us to answer questions in geometry just using algebra. It was this idea that made it possible for us to imagine the mathematical possibility of higher-dimensional spaces.

The questions below show how the idea of distance can be represented using algebra in coordinate geometry.

Once we know how to define distance, we can define a 4-dimensional sphere centered at 0 and of radius r to be the set of points in 4-dimensional space that are a distance r from the origin. This is the idea that is being used when we talk about the 8- and 24-dimensional sphere-packing problem.